“We depend on numbers to make sense of the world,
and have done so ever since we started to count.”
— Alex Bellos
***
The earliest symbols used for numbers go back to about 5000 years ago to Sumer (modern-day Iraq). They didn’t really look far for names. Ges, the word for one, also meant man. Min, the word for two, also meant women. At first, numbers served a practical purpose, mostly things like counting sheep and determining taxes.
“Yet numbers also revealed abstract patterns,” writes Alex Bellos in his fascinating book The Grapes of Math: How Life Reflects Numbers and Numbers Reflect Life, which, he continues, “made them objects of deep contemplation. Perhaps the earliest mathematical discovery was that numbers come in two types, even and odd: those that can be halved cleanly, such as 2, 4 and 6, and those that cannot, such as 1, 3 and 5.
Pythagoras and Number Gender
Pythagoras, the Greek teacher, who lived in the sixth century BC and is most famous for his theorem about triangles, agreed with the Sumerians on number gender. He believed that odd numbers were masculine, and even ones were feminine. This is where it gets interesting … why did he think that? It was, he believed, a resistance to splitting in two that embodied strength. The ability to be divisible by two, in his eyes was a weakness. He believed odd numbers were master over even. Christianity agrees with the gender theory, God created Adam first and Eve second. The latter being the sin.
Large Numbers
Numbers originally accounted for practical and countable things, such as sheep and teeth. Things get interesting as quantities increase because we don’t use numbers in the same way.
We approximate using a “round number” as a place mark. It is easier and more convenient. When I say, for example, that there were a hundred people at the market, I don’t mean that there were exactly one hundred people there. … Big numbers are understood approximately, small ones precisely, and these two systems interact uneasily. It is clearly nonsensical to say that next year the universe will be “13.7 billion and one” years old. It will remain 13.7 billion years old for the rest of our lives.
Round numbers usually end in zero.
The word round is used because a round number represents the completion of a full counting cycle, not because zero is a circle. There are ten digits in our number system, so any combination of cycles will always be divisible by ten. Because we are so used to using round numbers for big numbers, when we encounter a big number that is nonround— say, 754,156,293— it feels discrepant.
Manoj Thomas, a psychologist at Cornell University, argues that we are uneasy with large, non-round numbers, which causes us to see them as smaller than they are and carries with it practical implications when, say, selling a house. “We tend to think that small numbers are more precise,” he says, “so when we see a big number that is precise we instinctively assume it is less than it is.” If he’s right, the result is that you will pay more for expensive and non-round prices. Indeed his experiments seem to agree. In one, respondents viewed pictures of several houses and sales prices, some were round, and some were larger and non-round (e.g., $490,000 and $492,332). On average subjects judged the precise one to be lower. As Bellos concludes on large numbers, “if you want to make money, don’t end the price with a zero.”
Number Influence When Shopping
One of the ways to make a number seem more precise is by subtracting 1.
When we read a number, we are more influenced by the leftmost digit than by the rightmost, since that is the order in which we read, and process, them. The number 799 feels significantly less than 800 because we see the former as 7-something and the latter as 8-something, whereas 798 feels pretty much like 799. Since the nineteenth century, shopkeepers have taken advantage of this trick by choosing prices ending in a 9, to give the impression that a product is cheaper than it is. Surveys show that anything between a third and two-thirds of all retail prices now end in a 9.
Of course, we think that other people fall for this and surely not us, but that is not the case. Studies like this continue to be replicated over and over. Dropping the price one cent, say from $8 to $7.99 influences decisions dramatically.
Not only are prices ending in 9 harder to recall for price comparisons, but we’ve also been conditioned to believe they are discounted and cheap. The practical implications of this are that if you’re a high-end brand or selling an exclusive service, you want to avoid the bargain aspect. You don’t want a therapist who charges $99.99, any more than you want a high-end restaurant to list menu prices ending in $.99.
In fact, most of the time, it’s best to avoid the $ altogether. Our response to this stimulus is a pain.
The “$” reminds us of the pain of paying. Another clever menu strategy is to show the prices immediately after the description of each dish, rather than listing them in a column, since listing prices facilitates price comparison. You want to encourage diners to order what they want, whatever the price, rather than reminding them which dish is most expensive.
These are not the only nor most subtle ways that numbers influence us. The display of absurdly expensive items first creates an artificial benchmark. The real estate agent, who shows you a house way above your price range first, is really setting an artificial benchmark.
The $100,000 car in the showroom and the $10,000 pair of shoes in the shop window are there not because the manager thinks they will sell, but as decoys to make the also-expensive $50,000 car and $5,000 shoes look cheap. Supermarkets use similar strategies. We are surprisingly susceptible to number cues when it comes to making decisions, and not just when shopping.
We can all be swayed by irrelevant, random numbers, which is why it’s important to use a two-step framework when making decisions.
Numbers and Time
Time has always been counted.
We carved notches on sticks and daubed splotches on rocks to mark the passing of days. Our first calendars were tied to astronomical phenomena, such as the new moon, which meant that the number of days in each calendar cycle varied, in the case of the new moon between 29 and 30 days, since the exact length of a lunar cycle is 29.53 days. In the middle of the first millennium BCE, however, the Jews introduced a new system. They decreed that the Sabbath come every seven days ad infinitum, irrespective of planetary positions. The continuous seven-day cycle was a significant step forward for humanity. It emancipated us from consistent compliance with Nature, placing numerical regularity at the heart of religious practice and social organization, and since then the seven-day week has become the world’s longest-running uninterrupted calendrical tradition.
Why seven days in the week?
Seven was already the most mystical of numbers by the time the Jews declared that God took six days to make the world, and rested the day after. Earlier peoples had also used seven-day periods in their calendars, although never repeated in an endless loop. The most commonly accepted explanation for the predominance of seven in religious contexts is that the ancients observed seven planets in the sky: the Sun, the Moon, Venus, Mercury, Mars, Jupiter and Saturn. Indeed, the names Saturday, Sunday and Monday come from the planets, although the association of planets with days dates from Hellenic times, centuries after the seven-day week had been introduced.
The Egyptians used the human head to represent 7, which offers “another possible reason for the number’s symbolic importance.”
There are seven orifices in the head: the ears, eyes, nostrils and mouth. Human physiology provides other explanations too. Six days might be the optimal length of time to work before you need a day’s rest, or seven might be the most appropriate number for our working memory: the number of things the average person can hold in his or her head simultaneously is seven, plus or minus two.
Bellos isn’t convinced. He thinks seven is special, not for the reasons mentioned above, but rather because of arithmetic.
Seven is unique among the first ten numbers because it is the only number that cannot be multiplied or divided within the group. When 1, 2, 3, 4 and 5 are doubled the answer is less than or equal to ten. The numbers 6, 8 and 10 can be halved and 9 is divisible by three. Of the numbers we can count on our fingers, only 7 stands alone: it neither produces nor is produced. Of course the number feels special. It is!
Favorite Numbers and Number Personalities
When people are asked to think of a digit off the top of their head, they are most likely to think of 7. When choosing a number below 20, the most probable response is 17. We’ll come back to that in a second. But for now, let’s talk about the meaning of numbers.
Numbers express quantities, and we express qualities to them. Here are the results from a simple survey that paints a “coherent picture of number personalities.

Interestingly, Bellos writes, “the association of one with male characteristics, and two with female ones, also remains deeply ingrained.”
When asked to pick favorite numbers, we follow clear patterns, as shown below in a heat map, in which the numbers from 1 to 100 are represented by squares. Bellos explains:
(The top row of each grid contains the numbers 1 to 10, the second row the numbers 11 to 20, and so on.) The numbers marked with black squares represent those that are “most liked” (the top twenty in the rankings), the white squares are the “least liked” (the bottom twenty) and the squares in shades of gray are the numbers ranked in between.
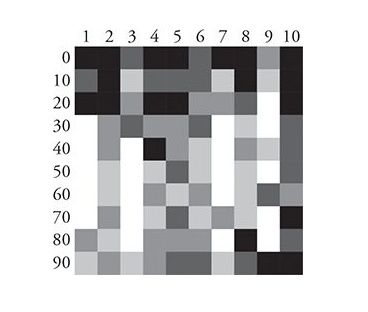
The heat map shows conspicuous patches of order. Black squares are mostly positioned at the top of the grid, showing on average that low numbers are liked best. The left-sloping diagonal through the center reveals that two-digit numbers where both digits are the same are also attractive. We like patterns. Most strikingly, however, four white columns display the unpopularity of numbers ending in 1, 3, 7 and 9.
Numbers are a part of our lives. We see them everywhere. They influence us, they guide us, and they help us solve problems. And yet, as The Grapes of Math: How Life Reflects Numbers and Numbers Reflect Life shows us, their history and patterns can also be a source of wonder.
